Distribution Stress Between Steel & Concrete

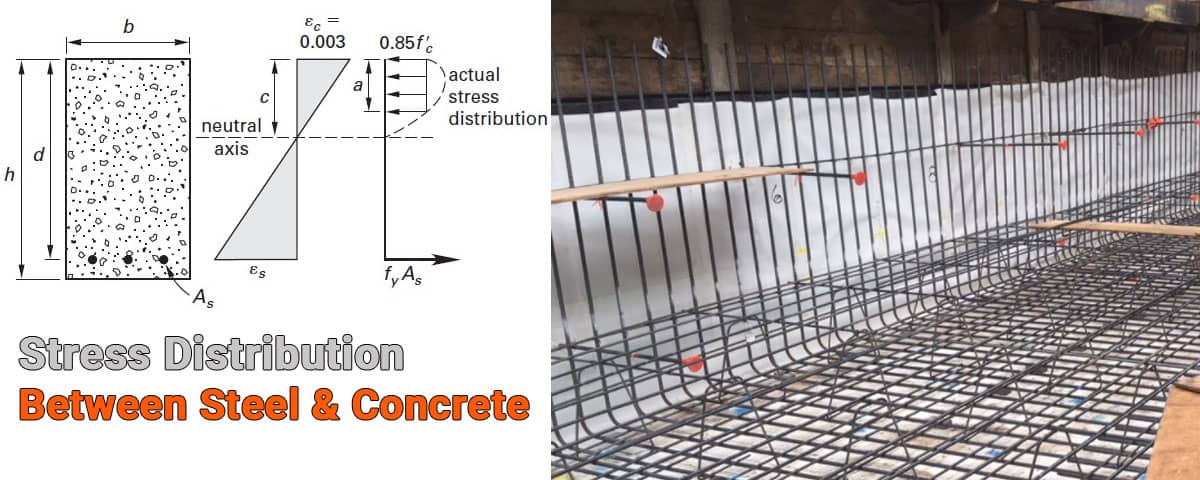
As per the essential assumption, plain RCC section before bending remains plain after bending. it's clear that within the flexural member at a selected point the compressive stress and therefore the tensile stress are proportional to their distance from N.A ( Neutral axis). because the bond between concrete and steel becomes excellent, strains induced in concrete also as within the steel are going to be equal.

Let strain in concrete and strain in steel be designated by ec and et

According to Hooke's law

Stress/Strain = Moduli of elasticity

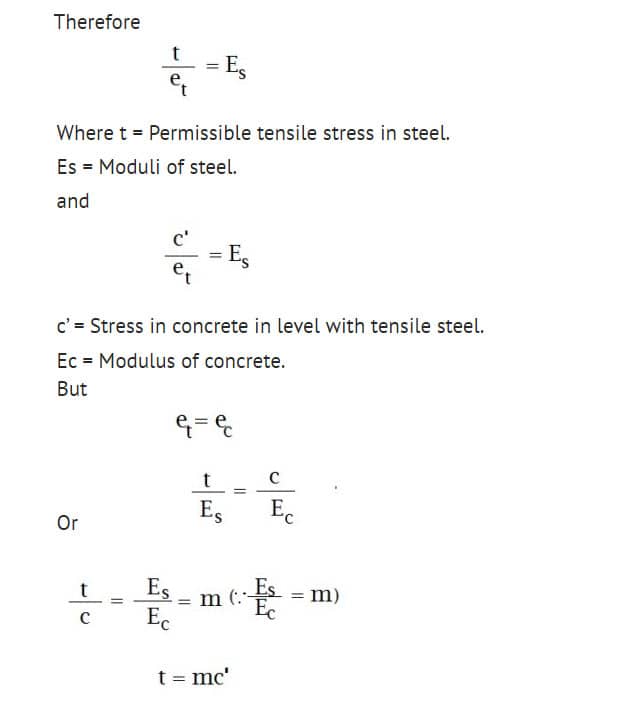
From the above expression, it's evident that the strain within the steel is m times the strain in concrete surrounding it.