Understanding the Various Types of Curves in Surveying
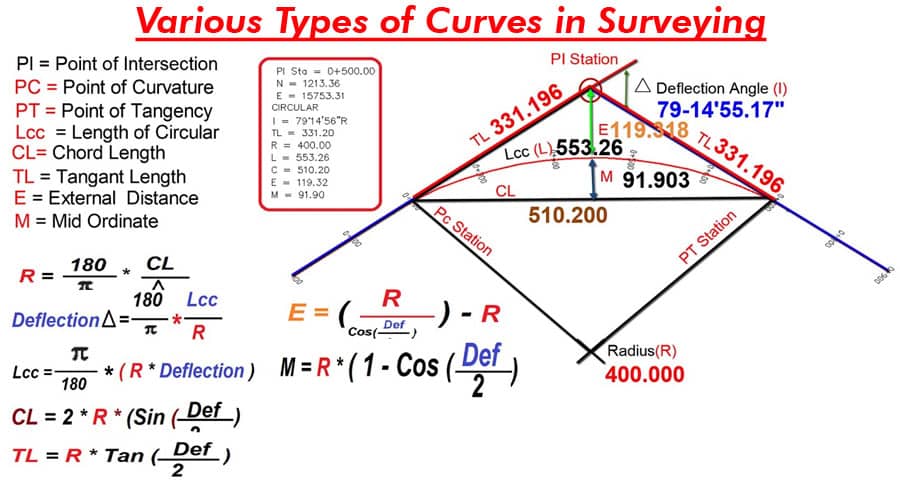
In the realm of surveying, a critical aspect that professionals encounter is the delineation and analysis of curves. These curves are essential components in the layout and design of infrastructure projects, ranging from highways and railways to pipelines and canals. Mastery over the different types of curves is indispensable for ensuring the efficiency, safety, and precision of surveying endeavors.
Circular Curves: A Foundation in Surveying
Circular curves represent the fundamental building blocks in surveying. These curves are characterized by their uniform curvature, resembling arcs of a circle. Surveyors frequently utilize circular curves in various applications, such as road alignments and railway tracks. Understanding the parameters of circular curves, including their radii and central angles, is crucial for achieving optimal alignment and smooth transitions in infrastructure projects.
Compound Curves: Enhancing Flexibility and Precision
In more complex surveying scenarios, the terrain or project requirements may necessitate the use of compound curves. Unlike simple circular curves, compound curves consist of multiple arcs with varying radii and directions. Surveyors employ compound curves to navigate challenging terrain, accommodate changes in alignment, and optimize the utilization of available space. Mastery over compound curves empowers surveying professionals to address intricate design requirements with precision and efficiency.
Spiral Curves: Achieving Seamless Transitions
Spiral curves represent a specialized category of curves utilized in surveying projects requiring smooth transitions between straight and curved sections. These curves are characterized by their gradually increasing curvature, enabling seamless integration between linear and curved alignments. Surveyors often incorporate spiral curves in highway design, railway tracks, and drainage systems to mitigate abrupt changes in alignment and enhance safety for users. Proficiency in designing and implementing spiral curves is essential for ensuring optimal functionality and user experience in infrastructure projects.
Transition Curves: Balancing Safety and Efficiency
In the domain of transportation engineering, transition curves play a pivotal role in achieving a delicate balance between safety and efficiency. These curves are strategically integrated between straight and circular segments to facilitate gradual changes in curvature, minimizing discomfort for travelers and enhancing vehicle stability. By carefully designing transition curves, surveyors can optimize sight distances, reduce vehicle wear and tear, and improve overall traffic flow. Understanding the principles governing transition curve design is essential for ensuring the safety and operational efficiency of transportation infrastructure.
Cubic Parabola Curves: Precision in Vertical Alignment
Beyond horizontal alignment, surveying professionals also encounter the challenge of optimizing vertical alignment to accommodate changes in elevation. Cubic parabola curves offer a sophisticated solution for achieving smooth transitions in vertical profiles, minimizing gradients, and maximizing user comfort. Surveyors utilize cubic parabola curves in applications such as road design, canal construction, and railway gradients to ensure optimal drainage, stability, and navigability. Proficiency in designing and implementing cubic parabola curves is paramount for optimizing the performance and longevity of infrastructure projects.
Elliptical Curves: Addressing Unique Geometric Challenges
In certain surveying scenarios, traditional circular or compound curves may not suffice to address the geometric intricacies of the terrain. Elliptical curves offer a versatile solution, allowing surveyors to accommodate irregularities in alignment while maintaining smooth transitions. These curves are characterized by their elongated shape, resembling ellipses, and provide greater flexibility in adapting to varying terrain profiles. Surveyors leverage elliptical curves in applications such as canal construction, where the path may deviate significantly from a straight line due to geological constraints or land use considerations.
Clothoid Curves: Optimizing Curvature Gradually
Clothoid curves, also known as Euler spirals, represent a specialized curve type utilized in surveying projects that require gradual changes in curvature along the alignment. Unlike traditional spiral curves, which feature a constant rate of curvature increase, clothoid curves offer a variable rate of change, allowing for smoother transitions between straight and curved sections. Surveyors often employ clothoid curves in railway track design, where precise control over curvature is essential to accommodate high-speed trains and ensure passenger comfort. Mastery over clothoid curve design enables surveying professionals to optimize track layouts, reduce maintenance costs, and enhance the operational efficiency of rail networks.
Bezier Curves: Precision in Digital Terrain Modeling
With the advent of digital technology, surveyors have access to advanced tools and techniques for terrain modeling and design. Bezier curves represent a key component in digital terrain modeling, enabling surveyors to represent complex surfaces with precision and efficiency. These curves are defined by control points that influence the shape and trajectory of the curve, allowing for greater flexibility in shaping terrain features such as contours, slopes, and embankments. Surveyors leverage Bezier curves in applications such as urban planning, environmental modeling, and infrastructure design to simulate real-world conditions accurately and optimize project outcomes.
Hyperbolic Curves: Addressing Extreme Geometric Constraints
In scenarios where traditional curve types may not suffice to address extreme geometric constraints, surveyors turn to hyperbolic curves as a viable solution. These curves, characterized by their asymptotic behavior, offer a unique means of navigating tight curves or abrupt changes in alignment. Surveyors deploy hyperbolic curves in applications such as tunnel design, where limited space and challenging geological conditions necessitate precise control over curvature and alignment. By mastering the principles governing hyperbolic curve design, surveying professionals can overcome geometric challenges and deliver innovative solutions that meet the needs of complex infrastructure projects.
For more information, please watch the following video tutorial
Source: Surveying Engineering Design Information
Conclusion
In conclusion, a comprehensive understanding of the various types of curves in surveying is indispensable for ensuring the success of infrastructure projects. From circular and compound curves to spiral and transition curves, each type serves a unique purpose in achieving optimal alignment, safety, and efficiency. By mastering the principles governing curve design and implementation, surveying professionals can navigate complex terrain, mitigate risks, and deliver exceptional results that meet the needs of stakeholders and communities.
